Mathematicians

Powerful Faces in Mathematics: Hypatia of Alexandria

This is the second post in a series on important women mathematicians in history. Click here to view the first post on Ada Lovelace who is often described as the first computer programmer.

Hypatia’s contributions involved several fields of mathematics including advanced geometry, computation methods, and applications to astronomy.

Let me begin with a coin question. Think about the steps you will use to find a solution.

Pretend you have $2.50 in dimes and quarters.

Hypatia Of Alexandria Dimes And Quarters

How many of each coin could you have?

Here are some observations you might make.

First: There are multiple possible number combinations.
Second: The answers are whole numbers. Fraction answers do not make sense.
Third: There are two unknowns; or, stating this another way, there is more than one variable.

Students might find the possible solutions by listing the pairs of values in a table. They might begin with all dimes (or all quarters) and then use trial and error. They may soon discover that the number of quarters must decease by 2 and the dimes increase by 5 to keep the total value equal to $2.50.

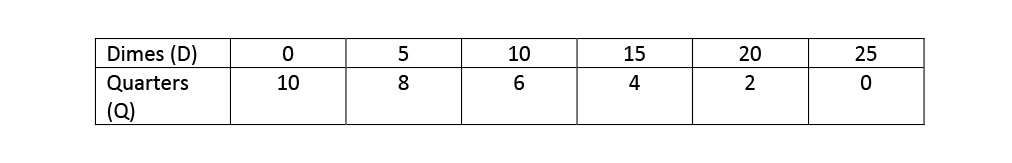
Microsoft Word Blog Images.docx

Another alternative is to use the variables in an equation such as 10•D + 25•Q = 250 using the values of the coins and the total expressed as cents. Equations like this were first proposed by Diophantus who lived in the 3^rdcentury. They are the beginnings of algebra. While Diophantus’ name is attached to these equations, another Greek mathematician arguably did more to expand on the ideas and ensure they were recorded for the future.

Hypatia of Alexandria 317-415 AD

In 2010, the UK’s Guardiannewspaper published a list of the “10 best mathematicians.” I found it interesting that the title of the list used the word “best” not “greatest” or “most well-known.” The list is presented in chronological order and begins with Pythagoras (circa 570-495 BCE). The second mathematician is Hypatia, a Greek woman who resided in Alexandria, Egypt, over 800 years later. This was a tumultuous time just before the start of the Dark Ages, when intellectual pursuits in the Eurocentric portion of the world ceased until about 1300 CE. As a result, the many accomplishments of Hypatia are too often overlooked and up until 20 years ago virtually undiscovered. It has been difficult to ascertain all that she did. However, recent scholarly works (Deakin, 2007) that have analyzed manuscripts indicate that the developments by Middle Eastern¹mathematicians, who were still highly active during the Dark Ages, refer to Hypatia’s accomplishments. It would seem that history focused on the negative aspects of that time rather than the positive developments from people such as Hypatia.

The Greeks achieved much in mathematics but generally relied on geometry to explore problems, provide proofs, and in general build knowledge they called mathema. They used pictures to prove that irrational numbers such as πand √2 existed, even though they did not have a method to record them. The Greeks squeezed a circle between many-sided polygons (inside and outside) to estimate the value of pi. But they did not have a broad mathematical language that included algebra.

Hypatia’s contributions involved several fields of mathematics including advanced geometry, computation methods, and applications to astronomy (Deakin, p 112). The most interesting and influential involves her work in algebra with a particular focus on problems related to Diophantine equations described above. The techniques developed for these equations allowed Greek mathematicians such as Hypatia to solve problems that were beyond the scope of geometry.

Until the last 20 years, the extent of Hypatia’s work involving algebra wasn’t clear. But analysis of Middle Eastern manuscripts indicates the style is that of Hypatia. It is known that she was a great teacher and did contribute much to recording the ideas of the time. Historians now feel that she added greatly to the advancements credited to others.

Endnotes:

The Pythagorean theorem was formalized and expressed as an equation by the Greeks. It is a Diophantine equation with exponents. During the Greek era, the lengths of sides of right triangles were restricted to whole number lengths that were passed down from the Sumerians. So, the solutions to equations were numbers such as 3,4,5; 6,8,10; 5,12,13, and so on.
The work with examples such as the coin problem are the basis for number theory. The correct number of dimes and quarters exist only for values that are the lowest common multiple of 10 and 25 or 0, 50, 100, 150, 200, 250, which in turn could be used to help find the solutions.
The 2009 movie Agora is about the life of Hypatia.

¹The expression Middle Eastern is used here to acknowledge that Persian and Arabic mathematicians collectively made many highly significant contributions to the development of algebra.

Deakin, Michael A.B. 2007. Hypatia of Alexandria. New York: Prometheus Books.