Mathematicians
Powerful Faces in Mathematics: Ada Lovelace
This is the first post in a series on important women mathematicians in history. Click here to view the second post on Hypatia of Alexandria who is described as being the first person to document mathematics.
In the history of machine computing there are only two computer languages named after people.
Those two are Blaise Pascal (1623–1662) and Ada Lovelace (1815–1852). Both inventors predate the era of electronic computing and are often overlooked due to the emphasis placed on 20th–century inventors. Pascal’s machine was a very simple device that could add by turning dials one way and subtract by turning in reverse. Lovelace’s contribution even more significant and she is correctly described as the first computer programmer.

Ada Lovelace
James Essinger’s 2013 book, A Female Genius, describes the life and contributions of Ada, the Countess of Lovelace. At her mother’s guidance, Ada studied arithmetic, music, and French. Her father, Lord Byron, influenced Ada’s interest in literature and poetry. Byron called his wife “the princess of parallelograms,” so it isn’t difficult to imagine that Ada’s mother was an influence on her daughter’s later mathematical pursuits.
The family was very active in the London social scene of the mid-1800s. At one of these events, Ada met Charles Babbage who shared his thoughts about a machine that could complete the tedious and often erroneous calculations in tables of data. Babbage had the idea for the machine and Ada saw how mathematics might be used to “program it.” Their initial efforts were intended to involve the preparation of accurate mathematical and astronomical tables as well as tide charts.
A machine could be used to calculate data for the tables that were related to patterns. In particular, predictable patterns generated by equations. The easiest patterns were mathematical tables of values from polynomials such as y= x2+ 3x – 6. Ada used what she knew and built on her mathematical knowledge of patterns to create the “programs.” At a basic level, the mathematics involved differences. As an example, consider the sequence of square numbers in the first row below that would arise from one of the simplest polynomials, y = x2. The pattern of square numbers is growing. The differences between the square numbers also grows but less quickly. The second sequence of differences is equal, or constant.

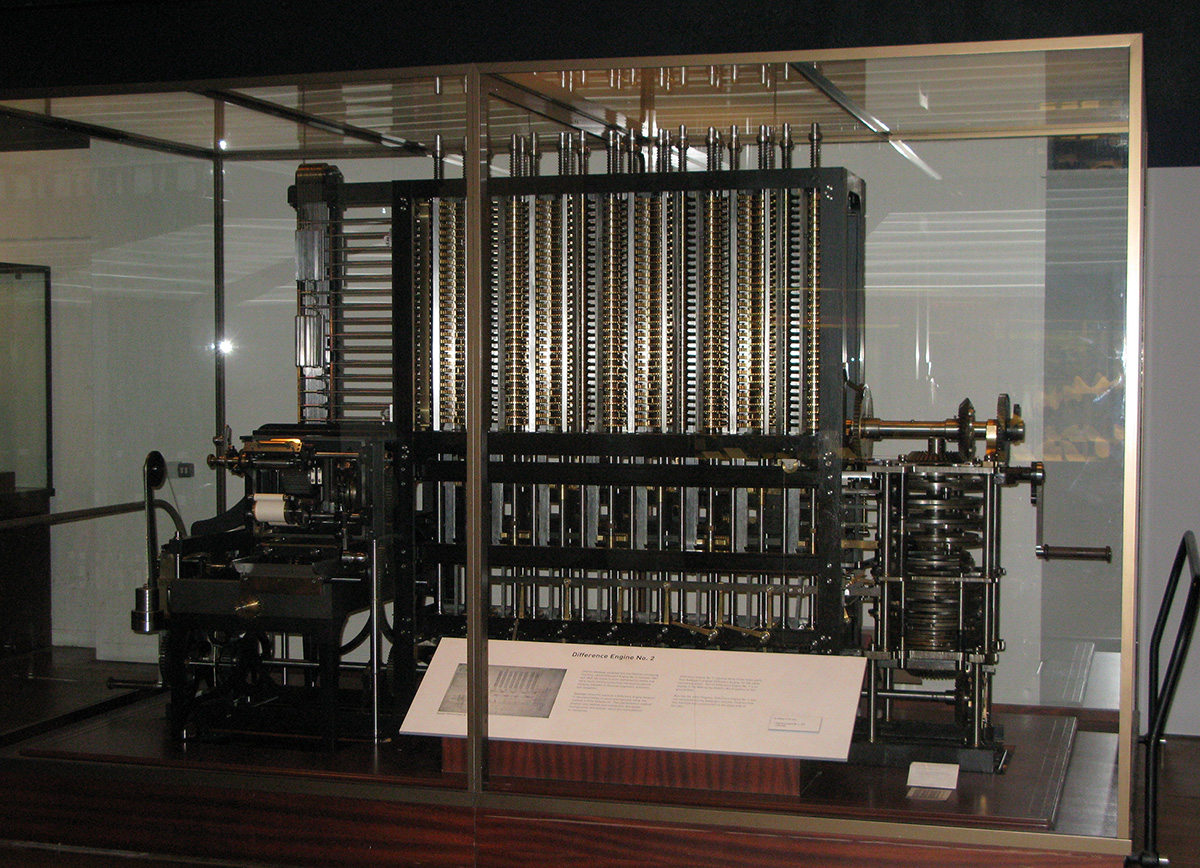
Babbage Difference Engine photo by user:geni, CC BY-SA 4.0, commons.wikimedia.org
Ada programmed the machines to begin with the first few values and then worked back from the constant differences to determine the values in the row of first differences and then the beginning row of square numbers. Ada had determined that constant differences at the second level meant the greatest value for an exponent in the polynomial was called 2. This called a polynomial of second degree.
Because the mathematical analysis involved differences, the first machine was called a Difference Engine. The above image shows the machine after it had finally been built and proven to beoperational. Babbage thought a machine should be able to do the calculations. But Ada was the person that analyzed and applied the necessary mathematics to enable it to work. The machine was never built during the 1800s, but it was constructed by the UK’s Science Museum in the 1990s and, in their words, “worked flawlessly.”
The Difference Engine wasn’t built because Ada developed further mathematical ideas so the pair started work on a more advanced computing machine called the Analytical Engine. This device could do more than work with differences and calculate values for complex equations.
Unfortunately, Ada died at the relatively young age of 36. It is interesting to theorize what might have happened if she had been able to make further contributions to mathematics.
Need a resource for additional math practice on patterns?
Consider The Algebra for All Series. This resource is a set of five books filled with teacher instructions and sequenced algebra activities.
This reasource for Grades 1-5 covers these concepts:
- Equivalence and Equations
- Properties
- Patterns and Functions
- Representations
Click here to download a Grade 3 activity from this series.




