Make a Change for Good: Part 3

Adopt a Piagetian approach to teaching mathematics concepts and skills

This article is the third of a five-part series on using what we know to modernize elementary math instruction:

• Part 1: Introducing influences in effective math instruction today
• Part 2: Implementing spaced learning
• Part 3: This post – Piaget theory in mathematics
• Part 4: Developing the language of mathematics
• Part 5: Fostering classroom discourse in math

Piaget Stages and A Piagetian Approach to Mathematics

Piaget is a name we don’t often read about these days. He proposed that children move through four stages of learning:

• His first stage (Sensorimotor) really only applied to children up to two years of age. But the next two stages are highly relevant to the elementary grades.
• In Stage 2 (Preoperational), Piaget suggested that children in the early grades of elementary school need concrete objects, pictures, actions, and symbols to develop mathematical meanings. For example, when teaching the “make-ten” addition strategy for figuring out basic facts such as 9 + 4, Grade 1 students should move counters on double ten-frames to act out the idea of using part of one addend to “make” the other addend into a complete “ten.” In this way they verbalize “nine plus four has the same value as ten plus three.” In doing so, numbers are treated as quantities, rather than symbols. At a later time, instruction is moved to a pictorial representation of the same problem. (Click here to learn more about this strategy.)

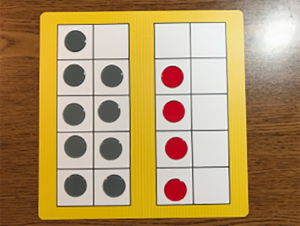
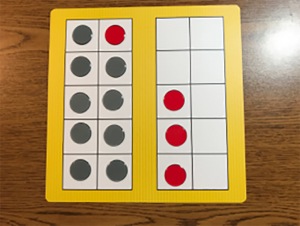
• Piaget’s Stage 3 (Concrete Operational) begins around Grade 2. In these grades students become more sophisticated in their thinking and begin to mentally visualize the concrete and pictorial manipulations as they apply them to more abstract problems. For example, if they have a strategy for 9 + 4, they can then continue to think quantitatively and apply the same thinking to 29 + 15. In the same way that “nine plus four has the same value as ten plus three,” then “twenty-nine plus fifteen has the same value as thirty plus fourteen.”
• The same thinking can be applied to 298 + 56, which removes the need to apply a traditional paper-and-pencil algorithm.

See this ORIGO ONE video for more on extending the make-ten addition strategy.

Teaching the make-ten (or bridge-to-ten) strategy for addition from ORIGO Education on Vimeo.

Not only does it make sense to use basic fact strategies as the foundation for computation with greater numbers, but due to Hattie’s study (see Part 1 of this series), we now know that this really works. The “effect” size of using this Piagetian approach is 1.28. As the average is 0.40, this represents more than three years of growth for each year of school! Perhaps it’s time to revisit Piaget’s classic thinking on how young children develop mathematical meanings and make it the mainstream approach to teaching mathematics in today’s classrooms.

Click here to read the next article in this series.