Learning to fish

Why learning math conceptually is important

I’m sure you’ve heard the saying, “Give a person a fish and you feed them for a day; teach a person to fish and you feed them for a lifetime.” Teaching math conceptually is something like that. Take multiplication, for instance. Yes, you could inflict the dreaded times tables on your students. If you learned your times tables to 12, as I did, you will recall there are 144 variations of this instrument of torture. This will not only fill your students with a dread of math for a lifetime, it will only give them the fish, not teach them how to fish.

Let’s take one equation from the 9 times table: 9 × 6 = 54 or “nine sixes are fifty-four.” You can have your students repeat this over and over like a mantra. They may memorize it so completely they’ll never forget it (I haven’t), but it will only ever give them the answer: 54. It is memorization without understanding; they have no idea how they got to 54.

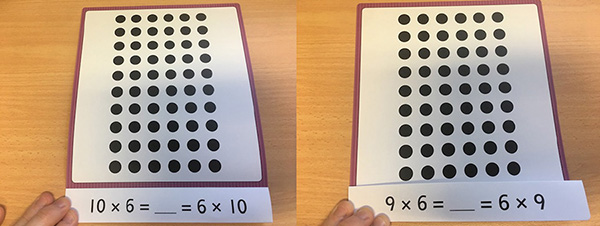
However, if you show your students on a visual model such an array (pictured below) that 9 × 6 is really the friendly expression of 10 × 6 less one row of 6, then it’s easy to arrive at 54. Ten rows of six is 60, less one row, and you get to 54. Most importantly, your students will know how they got there.

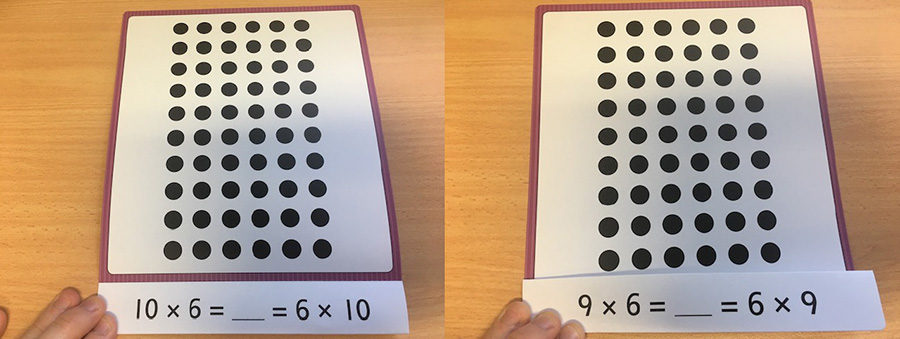
You can extend their understanding by rotating the array to show it does not change the total: 9 × 6 is the same as 6 × 9. Your students can see for themselves the commutative property of multiplication.

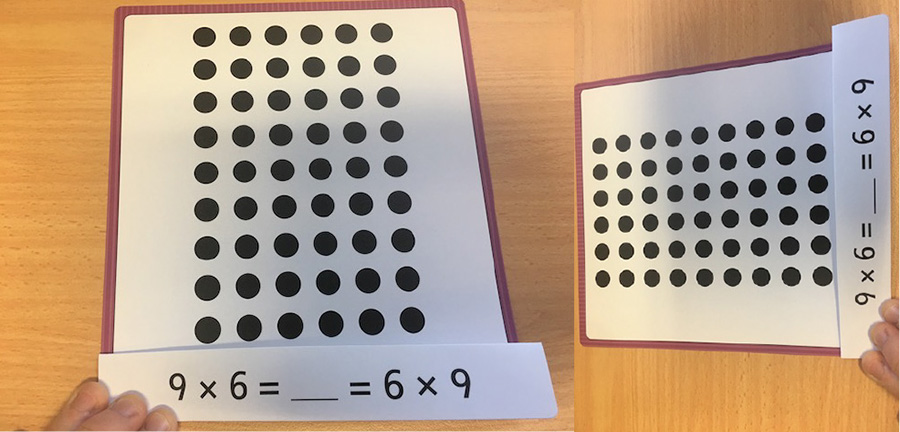
If kids only learn the times tables they’ll just learn 144 isolated pieces of information without appreciating the connections between those pieces. Conceptual math teaching shows them those connections.

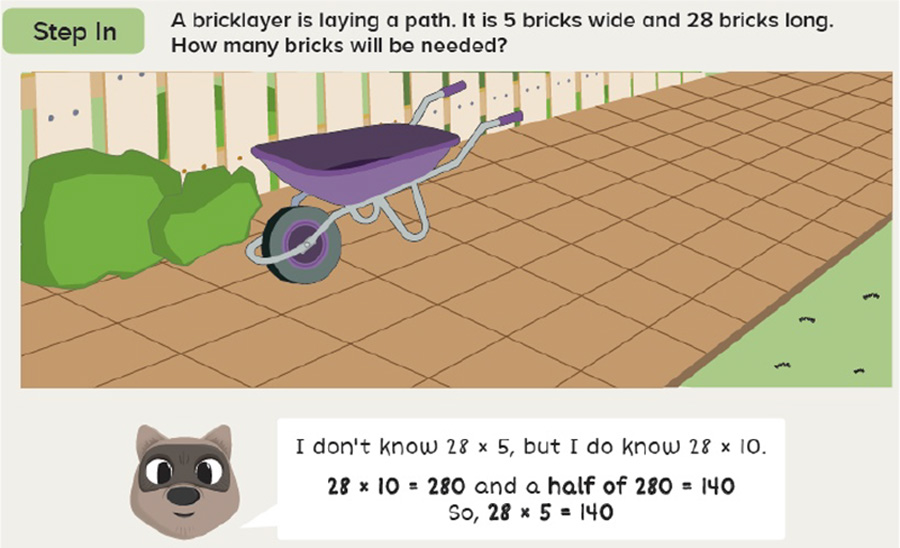
Let’s take another example. What’s 5 × 28? Skip counting by fives will get you there, but it’s painfully slow and not terribly efficient. So let’s use something kids know like the back of their hands: their ten fingers. Every kid knows the tens facts (1 ×10 is 10, 2 ×10 is 20, 3 ×10 is 30, and so forth). With a foldable array, you can show your students that the five facts are half of the tens facts. From that they can figure out, “Well, I don’t know five times 28 in my head. But ten times 28 is 280, so five times 28 must be half of that: 140.”

But it’s more than telling them, “Every time you see ‘multiply by five,’ you multiply by ten and halve it.” That’s just giving them another procedure they won’t understand. But if you show them an array they can visualize it. It’s the difference between giving and showing, between giving them the fish and showing them how to fish.

Parents, quite naturally, just want their kids to “get good grades.” Learning the times tables by rote may get your child a good grade. It may even get them an A. But you’re setting them up for an F later in life. Without solid number sense, without being comfortable around math, odds are they’ll carry this through to adulthood. Financial illiteracy — even something so simple as basic budgeting (Home Economics 101) — begins in childhood with a subliminal fear of numbers.

If kids get a sense of math they won’t fear it. They’ll feel comfortable around it. They won’t throw up their hands in resigned defeat and say, “I can’t do this.” And, it will help them in the long run. That’s the hidden benefit of conceptual math teaching — it keeps paying dividends well into adulthood.

Many adults, myself included, have a deep-seated fear of numbers. That’s because we were given the fish but never taught how to fish. A person who is given the fish must wait in hope for the next fish. If they’re not given one, they starve. A person who knows how to fish can fend for themselves.