Great Ideas
Make a Change for Good: Part 4
Develop students’ mathematical language to modernize elementary mathematics instruction
This article is the fourth of a five-part series on using what we know to modernize elementary math instruction:
- Part 1: Introducing 4 “influences or actions” on modern math instruction from ground-breaking research
- Part 2: Implementing spaced learning in mathematics
- Part 3: Adopt a Piagetian approach to teaching math concepts
- Part 4: How to develop mathematical language skills – this post
- Part 5: Fostering classroom discourse in the modern math classroom
We know from the study of neuroscience that in order to learn a new concept, we must connect the new idea to something we already know. Language is the tool we use to connect new ideas to existing ideas. In the previous article of this series, I wrote about the need to use a Piagetian approach to improve elementary math instruction. When a Piagetian approach is accompanied by the development of strong vocabulary, then it opens the classroom to richer discussions about math concepts.
Develop Students’ Mathematical Language
When you read equations such as 7 + 3 = 10 (“seven plus three equals ten”) and 7 – 3 = 4 (“seven minus three equals four”), you quickly realize that math has its own language. Unless children already have a solid understanding of the concepts behind these symbols, the words themselves are just abstract notions, devoid of any meaning—and children have no mental model to help them understand these terms.
Children are often introduced to abstract mathematical language far too early. As a result, many children struggle to grasp their meaning. We need to be very deliberate in the language we use to teach math concepts in the early grades, leveraging the words and ideas that children already know during instruction.
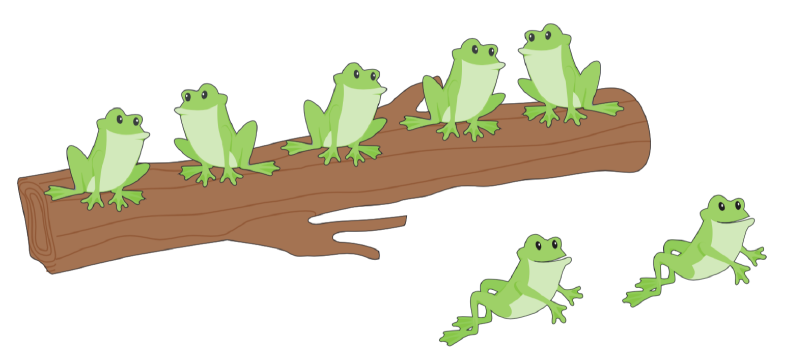
Very young children might not understand what words like “subtract” or “minus” mean, but they do know words that suggest or imply subtraction. For example, “I lost my toys.” “I ate the cupcakes.” “I broke an egg.” “I spent the money.” “The birds have flown away.” “The frogs jumped away.”
Stages of Math Language Development
In the first stage of development, which we call the student language stage, we use stories to stimulate discussion in the classroom. The stories make use of natural expressions (such as those above) that children are already know, to help them understand the concept of starting with a certain number of items and then taking some away.
In stage two, the materials language stage, we use hands-on, concrete resources that children can touch and manipulate to further develop their understanding. Typically, the teacher might ask a question such as: “If there are seven frogs on a log, and two jump off, how many are left?” The children would act out this scenario using counters, which helps them visualize the problem.
During this stage, the language greatly narrows from all that is available in the previous stage. Suddenly, the student can’t “eat” the blocks or counters. Just as they can’t easily lose, break, or spend them. And they can’t run away, fly away, or jump away either. With concrete materials, the language is reduced to “take away” or “cover up”. Similarly, with 2D drawings, we can only erase them or they can be crossed out.
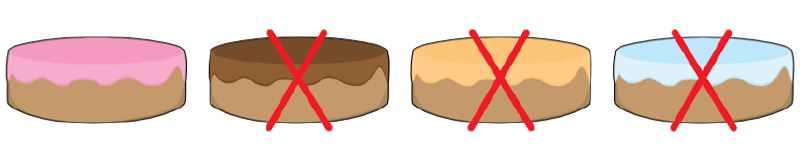
The third stage, the mathematical language stage, is where we introduce more precision with our language of math. Instead of saying “hop away” or “take away,” teachers would start using words like “subtract” or “minus,” while continuing to demonstrate this concept with the counters. Use a pocket chart, like the one shown below, to help children link the new language used during this stage to the language used in previous stages.

The final stage is the symbolic language stage. In this stage, teachers would introduce the subtraction symbol (–) as an abbreviation for all the words they have used before. If students are raced too fast to the symbolic language stage, there is a risk they have not had sufficient opportunities to build the required connections to deeply understand the meaning of subtraction.
See this ORIGO ONE video for more on developing mathematical language.
Introducing the language approach for teaching mathematics from ORIGO Education on Vimeo.
If you believe it makes sense to use language that children already know to help them build an understanding of new concepts, then it will come to no surprise that programs that aim to develop the language or “vocabulary” of mathematics have greater success. In fact, thanks to Hattie’s research (see Part 1 of this series), we now know that the effect size is 0.67. That’s more than a year and a half of growth for each year of schooling. Well worth the investment!
Click here to read the next article in this series.




